Are shaft vibration and unbalance limits too stringent for some rotors?

In the May/June 2015 issue of

Turbomachinery International

is an article “Myth: We really need more machinery standards” by Klaus Brun of Southwest Research Institute & Rainer Kurz of Solar Turbines. 

Many standards may be too long that require digital access for searching terms. Moreover, some specifications are too restrictive that adds unnecessary expense for all manufactures and thus cost for all.

As stated to the writer by one vendor: 

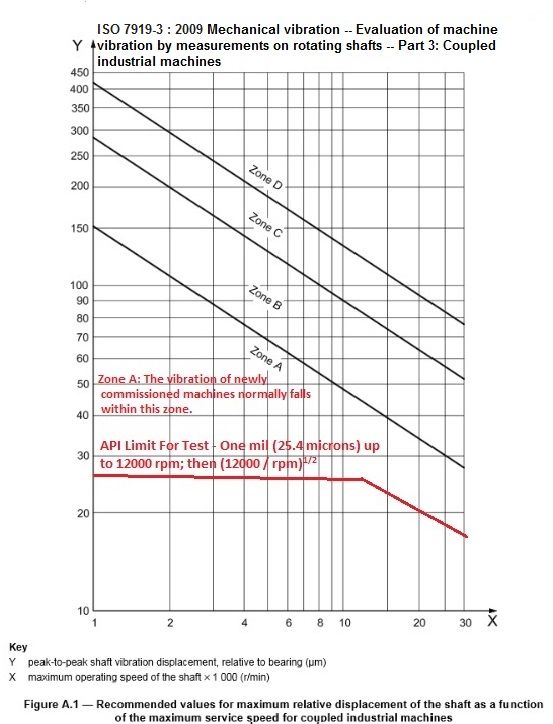
"While manufacturers are capable of meeting the existing vibration standards, tighter tolerances always come at a cost. The limits are unevenly applied due to a focus on 25 micrometers (one mil) displacement, which is at odds with how alarms and trips are set.  Field alarms and trips are based on a displacement that is inversely proportional to the square root of the speed per ISO 7919; in essence proportional to the square root of 12000/N. Therefore, the slower the speed, the higher the margin when leaving the factory."

The marked-up figure from ISO 7919 shows the disparity with the limit given in Reference 1, page 5-13, API standard paragraph SP6.8.8.8.

Three issues in the writer’s experience for API 617 (compressors) and API 612 (steam turbines) are:

• Limit of one mil (peak-peak) shaft displacement vibration is too low for low speed units especially with heavy rotors.
• Rotors balanced in low speed balance machines have low maximum limits, especially for low speed designs with heavy rotors.
• Unbalance distribution should be better allocated along the rotor for unbalance response calculations.

Shaft Vibration Limits:

Prior to the great advancement of eddy current shaft displacement probes (see USA expired 1964 U.S. Patent No. 3,316,756,"Vibration Monitoring System"), use of velocity limits at bearing housings was the norm. However there was much disparity in rotor to casing weight ratios and natural frequencies that resulted in poor decisions for shutdowns.

API standard paragraph from API 684 – reference 1 - limits shaft vibration as shown below.

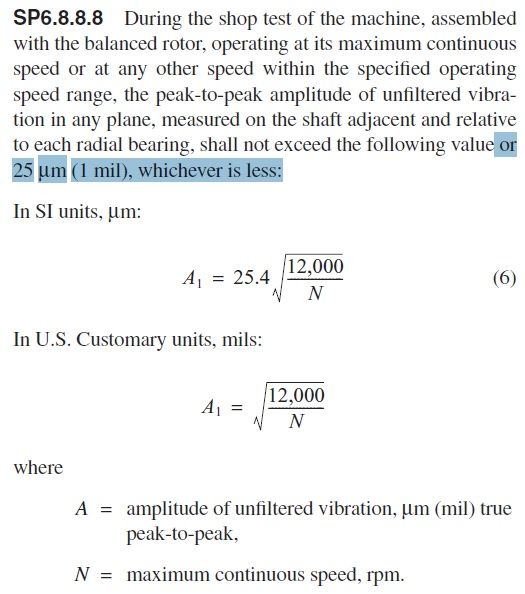
The original API specification for shaft vibration limit used the equation (12000 / RPM)1/2 and did not have the restriction:

“…or 25 µm, (1 mil), or whichever is less.”

Amplitude limit was lowered from two mils to one mil because of issues due to combinations of factors over the years, and users wanted low levels of vibration for plant start up issues. The original equation is also recommended by Perez and Conkey in Reference 2:

Perez and Conkey also note in reference 2: “Once a machine is installed in the field, a different vibration evaluation criterion is normally applied to take into account real-world effects, such as normal wear, piping strain, rotor fouling, etc.”

Vibration alarm and trip values in field operation are much higher for a machine running at 3600 rpm than for one at 12000 rpm. Thus a one mil limit during acceptance test or during initial field conditions is too restrictive for heavier rotors at lower speeds as shown below.

Dynamics of machinery should be based on velocity limits - not pure displacement. The relative dynamic loads on bearings and other components are also a function of speed. The original API equation, (12000 / rpm)1/2  at least gets closer to a velocity basis. For example, a machine running at 3600 rpm versus one running at 12000 rpm will have much lower relative dynamic loads and potential for seal rubbing with one mil vibration of the entire rotor:

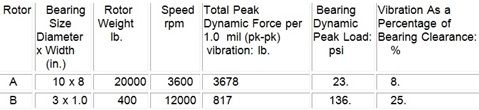
This assumes the entire rotor is vibrating at one mil (pk-pk) and rotor force is directly transmitted to the two bearings. In actuality rotor mode shapes / oil films will change dynamic load, but real unbalance response cases that calculate forces transmitted to bearings will give similar results.

Thus a compressor or turbine running at 3000 rpm with 2 mils vibration would be acceptable. An alternative to consider is one that a major oil company once specified:

For 0 to 3000 rpm  -  50 microns (2 mils)                                           

For 3000 to 15000 rpm  :  10 x (75000 / rpm)1/2 microns

e.g.  28.9 microns or 1.14 mils at 9000 rpm; 22.4 microns or 0.88 mils at 15000 rpm

For  > 15000 rpm  :  335,000 / rpm

e.g.  20.9 microns or 0.82 mils at 16000 rpm

Rotor Balance Limits

API's low-speed balance tolerances of 4W/N and that in ISO 1940 (reference 3) are in effect proportional to velocity, i.e. a function of speed). In other words, rotor mass eccentricity limit is a function of speed. ISO balance tolerance values such as Grade 2.5 for compressors and steam turbines is equivalent to an orbital velocity of the rotor center-of-gravity (2.5 mm/sec), calculated using the rotor eccentricity at operating speed. It is the writer’s opinion API went a little too far with value of 4W/N; they were likely trying to emulate Mil-STD-167, but that spec is based on total rotor weight, and is equivalent to 8W/N based on journal loads. Thus limit of 4W/N is probably reasonable for machines above 10,000 rpm but too conservative for those below 4000 rpm.

Low Speed example:

Rotor weight = 20,000 lb. :  max. continuous speed = 3,600 rpm

Journal Load = 10,000 lb.

4W/N = 11.1 in-ounce

 Mass eccentricity = (11.1/16) / 10,000 = 0.000070 inches (70 microinches)

 Velocity = 2 x Pi x f x d = 2 x 3.14 x (3600/60) x 0.000070 = 0.026 inches/sec (0.66 mm/sec)                                                                          

High speed example

Rotor weight = 2,000 lb. :  max. continuous speed = 10,000 rpm

Journal Load = 1000 lb.

4W/N = 0.4 in-ounce

Mass eccentricity = (0.4/16) / 1000 = 0.000025 inches ( 25 microinches)

Velocity = 2 x Pi x f x d = 2 x 3.14 x (10000/60) x 0.000025 = 0.026 inches/sec (0.66 mm/sec)

Thus ISO grade 2.5 low-speed balance value is still equivalent to 3.75 times the API tolerance of 4W/N, in other words is equal to 15W/N. This is deemed too high in the writer’s opinion unless the rotor is then high-speed balanced after an initial low-speed balance.

API 684 tutorial (reference 1) gives descriptions of benefits of high-speed balancing, along with cautions. The high-speed balance limits developed by the writer in the early 1980’s and currently suggested by API 684 are based on velocity of the pedestals. High-speed balance compensates for the true distribution of unbalance along the rotor, meeting a limit at maximum continuous speed but also considers the force at the first critical speed. After a high-speed balance the measurement in a low-speed balance machine often exceeds 4W/N.

Thus a suggested modification to the low speed balance limit (only for rotors without high-speed balancing) is as follows:

For 0 to 3000 rpm  :  8 W/N                                           

For 3000 to 6000 rpm  : 5.66 W/N x (6000 / rpm)1/2

For 6000 to 12000 rpm  :  4 W/N x (12000 / rpm)1/2

For  > 12000 rpm  :  4 W/N (Balance machine limitations occur near 25,000 rpm)

Granted the effective velocity of the rotor mass will still be required to be much lower comparing heavier low speed rotors to lighter high speed rotors, but machinery does not scale exactly relative to bearing loading, alternating stress, and encroachment of seal clearances.

Unbalance Distribution For Calculated Response

The API specifications also use multiples of unbalance in analytical unbalance response analysis. For example with twice allowable unbalance the calculated vibration is not to exceed the vibration limit over the operating speed range. Using the recommendations above would give more reasonable evaluations.

Another consideration for the next revision of API 684 is to change locations of unbalance for unbalance response analyses:

Instead of:

SP6.8.2.7

A separate damped unbalanced response analysis shall be conducted for each critical speed within the speed range of 0%–125% of trip speed. Unbalance shall analytically be placed at the locations that have been determined by the undamped analysis to affect the particular mode most adversely. For the translatory (symmetric) modes, the unbalance shall be based on the sum of the journal static loads and shall be applied at the location of maximum displacement. For conical (asymmetric) modes, an unbalance shall be added at the location of maximum displacement nearest to each journal bearing. These unbalances shall be 180 degrees out of phase and of a magnitude based on the static load on the adjacent bearing.

Suggested Revision:

A separate damped unbalanced response analysis shall be conducted for each critical speed within the speed range of 0%–125% of trip speed. Unbalance shall analytically be placed at the locations that are most likely to occur to affect the particular mode most adversely. For the translatory (symmetric) modes, the unbalance shall be based on the sum of the journal static loads and shall be distributed in phase along all the stages in the compressor or turbine. For conical (asymmetric) modes, an unbalance shall be added at the location of major elements at the ends of the rotor. These unbalances shall be 180 degrees out of phase and of a magnitude based on the static load on the adjacent bearing.

A third case would be with unbalance at the coupling(s) for use in unbalance response tests during mechanical testing.

References:

1. API RECOMMENDED PRACTICE 684 SECOND EDITION, AUGUST 2005 REAFFIRMED, NOVEMBER 2010

“API Standard Paragraphs Rotordynamic Tutorial: Lateral Critical Speeds, Unbalance Response, Stability, Train Torsionals, and Rotor Balancing; API Standard Paragraphs Rotordynamic Tutorial: Lateral Critical Speeds, Unbalance Response, Stability, Train Torsionals, and Rotor Balancing”

2. Perez, Robert, Conkey, Andrew, “Is My Machine OK: A Field Guide to Assessing Process Machinery”, Published: November, 2011, ISBN: 9780831134402, p. 143.

3. ISO 1940-1:2003, “Mechanical vibration -- Balance quality requirements for rotors in a constant (rigid) state -- Part 1: Specification and verification of balance tolerances

4. ISO 7919-3:2009, “Mechanical vibration -- Evaluation of machine vibration by measurements on rotating shafts -- Part 3: Coupled industrial machines”

Frank Kushner is an independent Consulting Engineer specializing in dynamics and acoustics testing and analysis. He has had 42 years of experience with industrial turbomachinery, mostly with the Elliott Company, and four years previous experience with the jet engine combustion section development group at Pratt & Whitney Aircraft. Mr. Kushner holds patents for a blade damping mechanism and a method to prevent a one-cell rotating stall in centrifugal compressors. frank_kushner@comcast.net